
[Contents] [Chapter I] [Chapter II] [Chapter III] [Chapter IV]
Mentula is a board game for four players. Although it is recommended to use a special Mentula board and pieces, it can be played with a chessboard and chess pieces as well.
Since the number of players is greater than two, coalitions may appear in the course of the game. During the fight of coalitions against each other, logic and strategy are widely used. When coalitions are created and destroyed, such phenomena as reliability or betrayal are manifested in the game. If more than one game is played among the same persons, some 'karmic' phenomena are simulated, too.
The other legend says that Mentula is an ancient Roman game, popular especially among prisoners who used to shout at jailers 'mentula! mentula!' excusing themselves that the abusive term was the name of the game they were just playing.
The fact is that the terms commonly used for some game situations (statura and doma) are Latin. Mentula itself is a Latin colloquial term meaning 'little dick'. Ceasar's officer Mamurra was mockingly called Mentula; Catullus mentions him under this name in four of his poems, for instance this distich:
Mentula moechatur. Moechatur Mentula? Certe!'Mentula is fornicating. Is Mentula fornicating? Surely! According to the proverb: by itself a cabbage pot gathers cabbage.' (Poem #94)
Hoc est quod dicunt: ipsa olera olla legit.
Reality was probably more prosaic. People who taught me Mentula had played it for the first time at The International Mathematical Olympiad in Warsaw, 1986. Since then, the game has occasionally been played and studied by Czech students of mathematics; I have no knowledge as to whether also elsewhere by anyone else.
In any case, the author of the game is unknown to me. Simplicity of the rules may indicate that the game is much older than 1986. I would be grateful if anybody could provide me any relevant information concerning the origin of the game.
As far as I know, this is the first (and till now the only one) Mentula page on the Internet. I am not aware of any book or article about Mentula either; please inform me if you are.
Mentula must have sometimes been played at the Board Games Club Paluba (Prague) as they mentioned it in their game list on a past version of their page
.My knowledge of Mentula originated in late 1980's with Adam Zach who then was a student of mathematics in Prague. A great deal of information presented here came to me from him. He has also made some research on the game (especially with one of the non-standard boards---the surface of the 4-dimensional regular 120-cell) and certainly has a lot of information unknown to me.
Mentula is played on a board of 8 times 8 squares of four colors which is called the mentuldesk or mentuldescu (see Origin of the Game). The four colors are used for convenience, but an ordinary chessboard may serve the purpose as well. At present, there is probably no manufacturer of Mentula boards and pieces, so the sets have to be home-made.
The squares of the metuldesk are colored this way:

(4*4 tiles of 2*2 playing squares of 4 different colors).
The role of the colors on a mentuldesk is similar to the role of the colors on a chessboard. On a chessboard, the bishop is bound to the squares of the same color due to the rules of its motion; similarly, the rules of Mentula force each of a player's pieces to stick to the squares of the same color.
There are no customary colors of the mentuldesk.
At the beginning of a game each player has four pieces of the same color. The usual starting position is this:
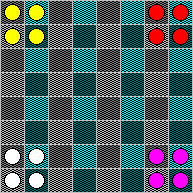
Some people, however, start with the following position:
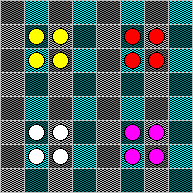
The latter position is used as the starting position on non-standard locally Euclidean boards.
There are no customary colors of pieces.
If a chess set is used for playing Mentula, each player takes four pieces of the same kind (usually four pawns, knights, bishops or rooks), two of them white and two black. They are placed on the chessboard so that the combination of the colors of pieces and squares simulates the four-colorness of the mentuldesk, for example this way:

Players take their moves one after another, usually clockwise. There is no customary starting color nor any fixed order of colors. It is useful to fix the order of colors for a game session and then draw lots.
The rule for moves is simple: Any of a player's pieces may jump over any other piece of the same player, moving to the square symmetrical with respect to the starting square, the center of symmetry being at the jumped-over piece. If the square is occupied by an opponent's piece, the opponent's piece is captured (removed from the board). The following picture shows examples of moves.
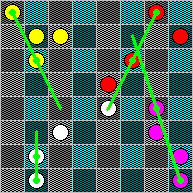
Notice that Red can capture a white piece. After making all these moves the position would become this:
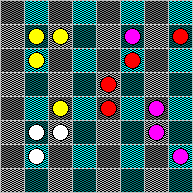
When a player cannot make any move, his turn is omitted. This happens whenever he has less than two pieces left, or when all pairs of his pieces aim off the board. Any such figure of player's pieces is called a statura. On the following diagram, all of the players are in a statura (note that such a situation can never happen in a real play).
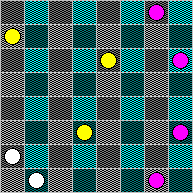
Notice that Red is in a statura because he has no pieces. Magenta is in a statura, though he has all four pieces left; this cannot happen in a real play, either: a player only falls into a statura position in consequence of loss of a piece.
Common algebraic notation known from chess can conveniently be used for recording Mentula positions and moves. The starting position may be denoted as
White (to play): a1, a2, b1, b2,
Yellow: a7, a8, b7, b8,
Red: g7, g8, h7, h8,
Magenta: g1, g2, h1, h2.
Moves are denoted in full by assigning the three squares that come into the move, for example a1-b3-c5 (or g5-f4xe3 for capturing). More shortly, only the destination square of the move may be put down, since any square can be reached by at most one piece of the player. (This, however, is not true for some of the non-standard boards; on such boards, at least the starting square and the destination square must be put down.) It is useful (though not necessary) to denote empty moves of the players in statura (eg. by a hyphen) to make clear who made the next turn.
This is an example of a record of the beginning of a game in full notation:
1. a1-b2-c3 b8-b7-b6 h8-g7-f6 g1-g2-g3In short notation, the same game would be recorded this way:
2. a2-c3-e4 b7-b6-b5 g8-f6xe4 g2-g3-g4
3. ...
1. c3 b6 f6 g3
2. e4 b5 e4 g4
3. ...
A player wins if all of his opponents have lost all of his pieces. This, however, happens rather rarely. Often one player is left with three pieces and another with one piece which is standing on a square of the former's missing color and thus is unreachable to him; or there may be two or more players left with mutually inaccessible couples of pieces. The situations of this kind have to be solved somehow, but none of the people that taught me Mentula were able to tell me how. If you have any suggestion (or any knowledge of a 'traditional' way of assigning the result to a game), please let me know.
A simple way of scoring is to assign each player as many points as the number of pieces he has at the end of the game. It seems to me, however, that a loss with one piece left is of much less worth than one third of a win with three pieces (or that to lose four games with one piece left is not better than a three piece win).
My way of scoring a game is therefore this: Each player is assigned the number of squares that he can reach, or rather cannot be denied to reach.
Thus a player whose opponents have lost all their pieces wins with the score of 64:0:0:0. A player left with three pieces against one piece of another player wins with the score of 48:1:0:0. In the position of the following diagram White wins 7:3:4:0. (Note that although White who is the first to move seemingly controls the e8 square, he will be denied by Red to reach it, being in zugzwang after Red makes his move; Red will then enter it safely.)
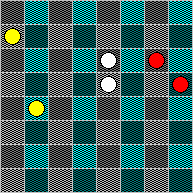
This way of determining the result seems fair and it allows a possibility of draw agreements if a coalition of players beats their opponents and its members do not want to proceed fighting now each other (of course, they would not be allowed to divide more points than the number of the squares they can reach altogether). On the other hand it is not clear if it can always be decided which squares are controlled by a player. It is even not clear when the game should stop, i.e. if it can always be proven that none of the players can increase his or decrease an opponent's score any more, or the ways players would agree on the fact that the game has ended and subsequently on the result. I'd be therefore grateful for any suggestions.
Making coalitions is an essential part of playing Mentula. There are two possible approaches to the question whether coalitions should be settled verbally or whether they should stem just from the game situation. Verbal arguing about allegiances is much more entertaining and a great deal of Mentula amusement lies in these arguments. Moreover the absence of any communication among the players can hardly be forced (and checked). Yet the silent game is also interesting, as it changes the tactics and strategy a lot. It is automatically performed whenever the players speak different languages or cannot talk with each other and so this style of play cannot be ignored. It seems to me that both styles are vital, the verbal one more fitting for friendly matches and the silent one for a tournament play.
[Contents] [Chapter I] [Chapter II] [Chapter III] [Chapter IV]